The discovery of ferroelectric properties in the doped HfO2 and mixed Hf1−xZrxO2 systems made precise phase determination very important. However, due to the similarities of the diffraction peaks between the tetragonal and the orthorhombic phases, the discrimination of these two critical phases by x-ray diffraction remains challenging. This work introduces Raman spectroscopy as a structural characterization method to unambiguously identify phases by comparing experimental data with density functional simulation results for the mixed hafnia–zirconia system in the complete composition range. Raman modes for the non-polar monoclinic and tetragonal phases are presented in comparison to those of the polar orthorhombic phase. Changes in phonon mode frequencies in the hafnia–zirconia system with Hf/Zr composition are related to the appearance of ferroelectric properties.
在掺杂 HfO2 和混合 Hf1−xZrxO2 系统中发现的铁电特性使得精确的相位测定变得非常重要。然而,由于四方相和正交相之间的衍射峰相似,通过 X 射线衍射区分这两个临界相仍然具有挑战性。这项工作介绍了拉曼光谱作为一种结构表征方法,通过将实验数据与整个成分范围内混合哈夫尼亚-氧化锆系统的密度泛函模拟结果进行比较来明确识别物相。非极性单斜相和四方相的拉曼模式与极性正交相的拉曼模式进行了比较。具有 Hf/Zr 成分的哈夫尼亚-氧化锆系统中声子模式频率的变化与铁电性质的出现有关。
I. INTRODUCTION I. 引言
Böscke et al. first described ferroelectricity in thin doped HfO2 films in 20111 and related the measured polarization hysteresis to the non-centrosymmetric polar orthorhombic III (o-) phase (Pca21). Further experiments by the same authors showed similar results in mixed oxides of hafnium and zirconium.2,3 In all cases, the polar phase appeared at the phase boundary between the monoclinic (m-) and tetragonal (t-) phases with space groups P21/c and P42/nmc, respectively. Introduction of dopants,4 optimized oxygen content,5 rapid quenching,6 high pressure,7 surface energy,8 mechanical stress,9 and electric field10 were suggested as reasons for the occurrence of the ferroelectric o-phase.11,12
Böscke 等人。2011 年首次描述了掺杂 HfO2 薄膜中的铁电性1,并将测得的极化滞后与非中心对称极性正交 III (o-) 相 (Pca2,1) 联系起来。同一作者的进一步实验表明,在铪和锆的混合氧化物中也有类似的结果。2,3 在所有情况下,极性相分别出现在空间群 P21/c 和 P42/nmc 的单斜晶相 (m-) 和四方相 (t-) 之间的相边界处。掺杂剂的引入、4 优化氧含量、5 快速淬火、6 高压、7 表面能、8 机械应力 9 和电场10 被认为是铁电 o 相出现的原因。11,12
Until now, most HfO2- or ZrO2-based thin films have been investigated by x-ray diffraction (XRD), since the method allows easy characterization of films in the nanometer range under small angles of incidence (GIXRD). Due to the structural similarity of the non-polar t- and polar o-phases, a strong overlap of the reflections is present, which makes it difficult to distinguish between the two phases. Minimal differences can also be caused by different mechanical stress in the films.13 This becomes particularly clear in the distinction of the main intensity peak in the range of 2θ values of about 30.6°. Here, the reflection of the polar o-phase in the (111) plane is at 2θ = 30.4° and that of the t-phase in the (101) plane is at 2θ = 30.8°.
到目前为止,大多数基于 HfO2 或 ZrO2 的薄膜都是通过 X 射线衍射 (XRD) 研究的,因为该方法可以在小入射角 (GIXRD) 下轻松表征纳米范围内的薄膜。由于非极性 t 相和极性 O 相的结构相似性,存在反射的强烈重叠,这使得很难区分这两个相。薄膜中的不同机械应力也可能引起微小的差异。13 这在区分约 30.6° 的 2θ 值范围内的主强度峰值时变得尤为明显。这里,(111) 平面中极性 o 相的反射为 2θ = 30.4°,(101) 平面中 t 相的反射为 2θ = 30.8°。
Raman spectroscopy (RS) is often used as another method for crystal phase characterization but has not been widely applied to HfO2 or ZrO2-based ferroelectric films. Similar to infrared spectroscopy, the assignment of vibrational modes allows the determination of the crystalline phases. The disadvantage compared to XRD is that thicker layers are typically required for Raman characterization. In the literature, one can find a classification of HfO2 and ZrO2 single crystals, ribbons, and powders showing m- and t-phase fractions under normal pressure conditions and leading to a transition to the orthorhombic-I phase (Pbca) under high pressures.14,15 For example, Ohtaka et al. described a transition from m- to orthorhombic-I-phase under high pressures for Y-doped ZrO2.16 Here, however, the anti-polar orthorhombic-I (Pbca) or non-polar orthorhombic-II (Pnma) phase was present, and the polar orthorhombic-III (Pbc21) was reported only at a later time. Accordingly, simulation results for this phase are also only recently available for undoped HfO217,18 or undoped ZrO2.19 Still, a detailed overview of the three phases (m-, t-, and oIII) for the complete Hf1−xZrxO2 composition range is missing.
拉曼光谱 (RS) 通常用作晶相表征的另一种方法,但尚未广泛应用于 HfO2 或 ZrO2 基铁电薄膜。与红外光谱类似,振动模式的分配允许确定晶相。与 XRD 相比,缺点是拉曼表征通常需要更厚的层。在文献中,可以找到 HfO2 和 ZrO2 单晶、带状和粉末的分类,在常压条件下显示 m 相和 t 相分数,并导致在高压下过渡到斜方 I 相 (Pbca)。14,15 例如,Ohtaka 等人描述了 Y 掺杂 ZrO2 在高压下从 m 相到正交 I 相的转变。16 然而,在这里,存在反极斜方晶 I (Pbca) 或非极性正方晶 II (Pnma) 期,而极方晶 III (Pbc21) 仅在较晚的时间报道。因此,该阶段的模拟结果也直到最近才可用于未掺杂的 HfO217,18 或未掺杂的 ZrO2。19 尽管如此,还缺少对整个 Hf1−x ZrxO2 成分范围的三个相(m-、t- 和 oIII)的详细概述。
In this work, Raman spectroscopy is used as a structural characterization method for the phase determination of thin Hf1−xZrxO2 films. Since an instability of an infrared active transverse optical mode occurs at a ferroelectric phase transition in Cochran's microscopic theory of ferroelectricity, Raman spectroscopy can be used to gain greater insight into the interplay of Raman active modes as the Hf1−xZrxO2 composition is changed. The unique Raman advantage to clearly separate slight structural differences between the o-phases and the t-phase, especially with respect to the position and bonding of the oxygen atoms, can be utilized to distinguish these phases. Furthermore, stress/strain is crucial in HfO2/ZrO2-based thin films for polar phase formation, and it can affect the Raman frequencies and intensities.17 Additional information might be gained by tracking phonon mode positions with Zr/Hf content. Here, mode crossings might give a hint about the origin of the ferroelectric properties in hafnia.
在这项工作中,拉曼光谱用作 Hf1−x ZrxO2 薄膜物相测定的结构表征方法。由于 Cochran 的铁电微观理论中,红外主动横向光学模式的不稳定性发生在铁电相变处,因此当 Hf1−x ZrxO2 成分发生变化时,拉曼光谱可用于更深入地了解拉曼主动模式的相互作用。拉曼光谱的独特优势可以清楚地区分 o 相和 t 相之间的微小结构差异,特别是在氧原子的位置和键合方面,可用于区分这些相。此外,应力/应变在基于 HfO2/ZrO2 的薄膜中对于极性相的形成至关重要,它会影响拉曼频率和强度。17 通过跟踪具有 Zr/Hf 含量的声子模式位置,可以获得更多信息。在这里,模式交叉可能暗示了哈夫尼亚中铁电性质的起源。
Since Raman determination can only be performed on films with a minimum thickness of about 30 nm, a film series with according thickness was fabricated. Furthermore, films with Al2O3 interlayers after every 10 nm of Hf1−xZrxO2 were also prepared to try to preserve the general crystal phase composition of 10 nm Hf1−xZrxO2 films as demonstrated for dynamic random access memory (DRAM)20 and ferroelectric capacitors.21 Density functional theory (DFT) calculations were used to simulate the Raman spectra in the complete Zr/Hf concentration range to compare with the experimental Raman data obtained from thin films. Both the simulations and the experimentally determined Raman spectra correlate well with the structural analysis obtained by GIXRD and with the electrically measured ferroelectric performance of the films, demonstrating the utility of the Raman technique applied to ferroelectric HZO. In the following text, only the polar orthorhombic-III phase is referred to as the o-phase.
由于拉曼测定只能在最小厚度约为 30 nm 的薄膜上进行,因此制造了具有相应厚度的薄膜系列。此外,还制备了在每 10 nm Hf1-xZrxO2 后具有 Al2O3 夹层的薄膜,以试图保持 10 nm Hf1-xZrxO2 薄膜的一般晶相组成,如动态随机存取存储器 (DRAM)20 和铁电电容器所示。21 密度泛函理论 (DFT) 计算用于模拟整个 Zr/Hf 浓度范围内的拉曼光谱,以与从薄膜获得的实验拉曼数据进行比较。模拟和实验确定的拉曼光谱都与 GIXRD 获得的结构分析以及薄膜的电测量铁电性能密切相关,证明了拉曼技术应用于铁电 HZO 的实用性。在下文中,只有极地正交 III 相被称为 o 相。
II. MATERIALS AND METHODS
二、材料与方法
A. Experimental section A. 实验部分
Capacitors were fabricated on top of (100) oriented p-doped silicon wafer substrates. As the first step, an 80 nm thick tungsten (W) layer was deposited via sputtering (PVD) in an Alliance Concept sputtering tool at 100 W target power and 30 SCCM Ar flow. Afterwards, a 10 nm titanium nitride (TiN) layer was sputtered in a Bestec ultrahigh vacuum cluster at room temperature using a Ti target and N2 plasma. The deposited TiN layer served as the bottom electrode (BE). After TiN BE deposition, atomic layer deposition (ALD) at 280 °C was performed to grow a 30 nm stack consisting of Hf1-xZrxO2. The film composition was varied by modifying the Hf to Zr precursor cycle ratio for a specific number of ALD cycles, referred to as a supercycle and defined here as Zr/(Zr + Hf). This fabrication step proceeded according to two process variations. For the first set of samples, continuous 30 nm Hf1−xZrxO2 layers were deposited using Tetrakis[ethylmethylamino]hafnium [Hf[N(C2H5) CH3]4] and Tris(dimethylamino) cyclopentadienyl-Zirconium (C5H5)Zr[N(CH3)2]3 as precursors for Hf, and Zr, respectively. For the second set of samples, an additional interlayer of five cycles of Al2O3 after every 10 nm of Hf1-xZrxO2 was added using trimethylaluminum (TMA) as a precursor keeping the total film thickness at approximately 30 nm. In both cases, O3 gas was used as the oxidant applied at a volume flow rate of 700 SCCM with a density of 150 g m−3. After ALD, a 10 nm TiN top electrode (TE) was deposited through a shadow mask in the Bestec chamber to realize patterned top electrodes. A 20 s rapid thermal anneal at 500 °C in an N2 atmosphere was employed to achieve crystallization of the Hf1−xZrxO2 dielectric layer. However, 500 °C was not enough to crystallize the capacitor structures made of undoped hafnium oxide containing alumina interlayers, therefore, the HfO2 with alumina interlayers stack had to be annealed at 700 °C for 20–40 s to achieve crystallization. The samples fabricated with the process described above were used for electrical and structural characterization as well as GIXRD measurements. However, to get a stronger signal during Raman measurements, an SC-1 etch of the TiN TE was also employed using H2O, H2O2, and NH3 at a 50:2:1 ratio for 1 min at 50 °C to reduce the TiN thickness from approximately 10 to 5 nm.
电容器是在 (100) 定向 p 掺杂硅晶片衬底上制造的。第一步,在 Alliance Concept 溅射工具中以 100 W 靶材功率和 30 SCCM Ar 流量通过溅射 (PVD) 沉积 80 nm 厚的钨 (W) 层。然后,在室温下使用 Ti 靶材和 N2 等离子体在 Bestec 超高真空簇中溅射 10 nm 氮化钛 (TiN) 层。沉积的 TiN 层用作底部电极 (BE)。TiN BE 沉积后,在 280 °C 下进行原子层沉积 (ALD),以生长由 Hf1-xZrxO2 组成的 30 nm 堆栈。通过修改特定数量的 ALD 循环(称为超级循环,此处定义为 Zr/(Zr + Hf))的 Hf 与 Zr 前驱体循环比率来改变薄膜组成。该制造步骤根据两种工艺变化进行。对于第一组样品,使用四[乙基甲基氨基]铪[Hf[N(C2H5) CH3]4]和三(二甲氨基)环戊二烯基锆(C5H5)Zr[N(CH3)2]3作为Hf和Zr的前驱体,分别沉积连续的30 nm Hf1−xZrxO2层。对于第二组样品,使用三甲基铝 (TMA) 作为前驱体,在每 10 nm 的 Hf1-xZrxO2 后添加一个额外的 Al2O3 循环夹层,保持总膜厚约为 30 nm。在这两种情况下,都使用 O3 气体作为氧化剂,体积流速为 700 SCCM,密度为 150 g m-3。 ALD 后,通过 Bestec 腔室中的阴影掩模沉积 10 nm TiN 顶部电极 (TE),以实现图案化顶部电极。在 N2 气氛中,在 500 °C 下进行 20 秒的快速热退火,以实现 Hf1−x ZrxO2 介电层的结晶。然而,500 °C 不足以使含有氧化铝中间层的未掺杂氧化铪制成的电容器结构结晶,因此,带有氧化铝中间层叠层的 HfO2 必须在 700 °C 下退火 20-40 秒才能实现结晶。使用上述工艺制造的样品用于电气和结构表征以及 GIXRD 测量。然而,为了在拉曼测量过程中获得更强的信号,还使用 H2O、H2O2 和 NH3 在 50 °C 下以 50:2:1 的比例对 TiN TE 进行 SC-1 刻蚀 1 分钟,以将 TiN 厚度从大约 10 nm 降低到 5 nm。
Sample thicknesses were measured by x-ray reflectivity with a Bruker D8 Discover XRD tool using a Cu Kα source. The crystalline phase was estimated via grazing incidence angle x-ray diffraction (GIXRD) on the same tool. Raman measurements were carried out at room temperature with a Raman microscope (Renishaw inVia Qontor), using a 457 nm wavelength laser, 100× objective lens, and 5000 s total measurement time per spectrum. Polarization vs electric field (P-E) hysteresis measurements were performed with an aixACCT Systems TF Analyzer 3000 using a measurement frequency of 1 kHz, whereas cycling was conducted at a frequency of 100 kHz.
使用 Cu Kα源,使用布鲁克 D8 Discover XRD 工具,通过 X 射线反射率测量样品厚度。在同一工具上通过掠入角 X 射线衍射 (GIXRD) 估计结晶相。在室温下使用拉曼显微镜 (Renishaw inVia Qontor) 使用 457 nm 波长激光器、100× 物镜进行拉曼测量,每个光谱的总测量时间为 5000 s。使用 aixACCT Systems TF Analyzer 3000 使用 1 kHz 的测量频率进行极化与电场 (P-E) 磁滞测量,而循环以 100 kHz 的频率进行。
B. Theoretical section B. 理论部分
First-principles calculations were performed using the ABINIT implementation of the density functional theory (DFT) and the density functional perturbation theory (DFPT) using the local density approximation (LDA) and projector augmented wave (PAW) pseudopotentials from the GBRV library. The pseudopotentials contain two partial waves for each s, p, and d channel for Zr, and two partial waves for each s, p, d, and f channel for Hf, providing high accuracy. The plane wave cut-off was 40 Ha, and the PAW cut-off 45 Ha. For the 12-atomic structures, the reciprocal space was sampled with a mesh of size 6 × 6 × 6. The force criterion for the atomic relaxation was better than 10−4 eV/Å, and the structures were fully relaxed.
使用来自 GBRV 库中的局部密度近似 (LDA) 和投影仪增强波 (PAW) 赝势,使用密度泛函理论 (DFT) 的 ABINIT 实现进行密度泛函理论 (DFT) 和密度泛函扰动理论 (DFPT) 进行第一性原理计算。赝势包含 Zr 的 s、p 和 d 通道的两个部分波,以及 Hf 的 s、p、d 和 f 通道的两个部分波,精度高。平面波边界值为 40 公顷,PAW 边界为 45 公顷。对于 12 原子结构,使用尺寸为 6 × 6 × 6 的网格对倒易空间进行采样。原子弛豫的力准则优于 10−4 eV/Å,并且结构完全松弛。
Phonons were calculated at the Gamma-point. The Raman tensor was computed using third-order DFPT. The longitudinal optical–transverse optical mode splitting (LO-TO) from long-range electrostatic interaction was considered with the analytical formula implemented in ABINIT. The Raman frequency of an oriented crystal depends on the direction q of the phonon. The Raman intensity of an oriented crystal depends on the frequency of the incident laser and the temperature of the sample. In a polycrystalline sample, the summation over all possible orientations in the Placzek approximation22 leads to an algebraic expression containing the rotational invariants G(0), G(1), and G(2) of the Raman tensor. Furthermore the average over all phonon direction q is done. The final spectra were calculated with a Lorentzian function using a broadening of 3 cm−1.
声子是在 Gamma 点计算的。拉曼张量是使用三阶 DFPT 计算的。使用在 ABINIT 中实现的分析公式来考虑来自长程静电相互作用的纵向光学-横向光学模式分模 (LO-TO)。取向晶体的拉曼频率取决于声子的方向 q。取向晶体的拉曼强度取决于入射激光的频率和样品的温度。在多晶样品中,Placzek 近似22 中所有可能方向的总和导致包含拉曼张量的旋转不变量 G(0)、G(1) 和 G(2) 的代数表达式。此外,所有声子方向 q 的平均值已完成。最终光谱是用洛伦兹函数计算的,展宽为 3 cm-1。
The investigated structures were based on 12-atomic representations of the o-phase (Pca21), t-phase (P42/nmc) and m-phase (P21/c) in Hf1−xZrxO2. These structures allow a representation for Zr-ratio x = 0 (HfO2), x = 0.25 (Hf0.75Zr0.25O2), x = 0.5 (Hf0.5Zr0.5O2), x = 0.75 (Hf0.25Zr0.75O2), and 1.0 (ZrO2). Two (three) inequivalent structures are present for different arrangements of Zr and Hf in Hf0.75Zr0.25O2 and Hf0.25Zr0.75O2 (Hf0.5Zr0.5O2). The structures were all relaxed, and the resulting Raman spectra were averaged. As a consequence, the spectra are broadened, and a strict assignment of a mode frequency to a mode symmetry is not possible for mixtures of Hf and Zr. Larger supercells with more possible Hf and Zr arrangements for a given composition would show even more broadening.
研究的结构基于 Hf1−x ZrxO2 中 o 相 (Pca21)、t 相 (P42/nmc) 和 m 相 (P21/c) 的 12 原子表示。这些结构允许表示 Zr 比 x = 0 (HfO2)、x = 0.25 (Hf0.75Zr0.25O2)、x = 0.5 (Hf0.5Zr0.5O2)、x = 0.75 (Hf0.25Zr0.75O2) 和 1.0 (ZrO2)。对于 Zr 和 Hf 的不同排列,在 Hf0.75、Zr0.25O2 和 Hf0.25、Zr0.75O2 (Hf0.5、Zr0.5O2) 中存在两(三个)不等等结构。这些结构都是松弛的,得到的拉曼光谱被平均。因此,光谱被展宽,并且对于 Hf 和 Zr 的混合物,不可能将模式频率严格分配给模式对称性。对于给定的成分,具有更多可能的 Hf 和 Zr 排列的更大超级单元将显示更多的展宽。
For experiments with changing composition, it would be interesting and relevant to be able to follow the same modes from ZrO2 to HfO2, if feasible. To explore this possibility, the modes for HfO2 and ZrO2 were classified from the symmetry of the calculated Raman tensor using the tables in the Bilbao crystallographic server,23,24 details can be found in the supplementary material. For example, for the polar o-phase, two symmetric mode symmetries A1, A2 and two antisymmetric mode symmetries B1, B2 (Mulliken symbols) are found.
对于改变成分的实验,如果可行,能够遵循从 ZrO2 到 HfO2 的相同模式将是有趣且相关的。为了探索这种可能性,使用毕尔巴鄂晶体学服务器中的表格根据计算的拉曼张量的对称性对 HfO2 和 ZrO2 的模式进行分类,23,24 详细信息可以在补充材料中找到。例如,对于极性 o 相,可以找到两个对称模式对称性 A1、A2 和两个反对称模式对称性 B1、B2(Mulliken 符号)。
The frequencies of the same modes increase from ZrO2 to HfO2 for large wave-numbers but decrease for small wave-numbers. For the mixed composition HZO, the supercell symmetry is reduced and the different atomic arrangement lead to different mode frequencies. The symmetry of the modes can still be identified investigating the symmetry of the Raman tensor23,24 and the mode indices can be assigned because the perturbation of the mode frequencies from the mass difference of Hf and Zr is moderate and depends continuously on composition. After the mode assignment, frequencies of the same modes are found to differ for inequivalent HZO atomic arrangements (see Table 2.2 in the supplementary material), which causes a stoichiometric broadening of mode frequencies. But, the averaged spectrum of the inequivalent atomic arrangements still shows the continuous dependence on stoichiometry. We expect for larger supercells with an increasing number of inequivalent configurations of the same stoichiometry, which better approximates a solid solution, a perfectly smooth dependence of the spectrum on stoichiometry.
对于数,相同模式的频率从 ZrO2 增加到 HfO2,但对于小波数,频率降低。对于混合成分 HZO,超胞对称性降低,不同的原子排列导致不同的模式频率。研究拉曼张量23,24 的对称性仍然可以确定模式的对称性,并且可以分配模式索引,因为 Hf 和 Zr 质量差异对模式频率的扰动是适中的,并且持续取决于成分。在模式分配之后,发现相同模式的频率对于不等效的 HZO 原子排列不同(参见补充材料中的表 2.2),这会导致模式频率的化学计量展宽。但是,不等价原子排列的平均谱仍然显示出对化学计量的连续依赖性。我们预计更大的超级单元具有越来越多的相同化学计量的不等价构型,这更接近固体解,即光谱对化学计量的完全平滑依赖性。
For the ferroelectric polar o-phase, the mode assignment is further complicated due to the LO–TO splitting, which lifts the degeneracy of the Raman mode frequencies. As expected for this high-k material, the mode splitting is relatively large. For the non-polar t-phase and m-phase, the Raman active modes show no LO–TO splitting, which makes the mode assignment easier and keeps the number of modes limited for these two phases.
对于铁电极 o 相,由于 LO-TO 分裂,模式分配变得更加复杂,这提高了拉曼模式频率的简并性。正如预期的那样,对于这种高 k 材料,模式分裂相对较大。对于非极性 t 相和 m 相,拉曼有源模式没有 LO-TO 分裂,这使得模式分配更容易,并保持了这两个相的模式数量限制。
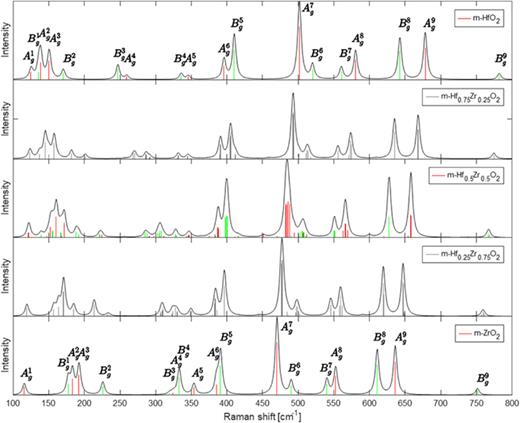
Figure 1 shows the calculated Raman intensities for the t-phase. The active modes are23,24 A1g + 2B1g + 3Eg and counted with increasing wave-number. The dominant mode is the symmetric
图 1 显示了计算出的 t 相拉曼强度。有源模式为23,24 A1g + 2B1g + 3Eg,并随着波数的增加而计数。主导模式是对称的,它随构图变化不大。
Raman intensities for the t-phase (P42/nmc). The active modes are23 A1g + 2B1g + 3Eg and labeled with increasing wave-number.
The Raman intensities for the polar o-phase are presented in Fig. 2. The 33 active modes are23,24 8A1 + 9A2 + 8B1 + 8B2, and only the most intense modes are labeled. The bar on the Mulliken index indicates a TO mode, otherwise, it is an LO mode. The dominant modes of this phase are the
极性 o 相的拉曼强度如图 2 所示。33 种活动模式是23,24 8A1 + 9A2 + 8B1 + 8B2,并且只标记了最强烈的模式。Mulliken 指数上的条形表示 TO 模式,否则为 LO 模式。该相的主要模式是
For HfO2, the Raman modes and intensities have previously been calculated.17,18 A comparison of the results shows good general agreement and agreement in the position of the signature modes being at 353/342/354 cm−1, 397/390/395 cm−1, 500/490/497 cm−1, and 565/555/560 cm−1 according to our own calculations and from Refs. 17 and 18. Figure 3 depicts the Raman intensities for the m-phase. 18 active modes are23,24 9 Ag + 9 Bg, which are all labeled. The most dominant mode is the
对于 HfO2,拉曼模式和强度之前已经计算过。17,18 结果的比较表明,根据我们自己的计算和参考文献,签名模式位于 353/342/354 cm-1、397/390/395 cm-1、500/490/497 cm-1 和 565/555/560 cm-1 的位置具有良好的总体一致性和一致性。17 和 18.图 3 描述了 m 相的拉曼强度。18 种主动模式是23,24 9 Ag + 9 Bg,它们都被标记了。最主要的模式是 500 cm-1 以下的模式。
Raman intensities for the polar o-phase (Pca21): 33 active modes are23 8A1 + 9A2 + 8B1 + 8B2, only the most intense modes are labeled.
Raman intensities for the m-phase (P21/c): 18 active modes are23 9Ag + 9Bg which are all labeled.
Superposition of the Raman spectra of the three different phases for different Zr/Hf compositions.
不同 Zr/Hf 成分的三种不同相的拉曼光谱叠加。
Superposition of the Raman spectra of the three different phases for different Zr/Hf compositions.
III. RESULTS AND DISCUSSION
III. 结果与讨论
Typically, the best ferroelectric properties in Hf1−xZrxO2 layers are reported in a film thickness range between 5 and 25 nm.25 Since the Raman intensity is very weak in this thickness range, a layer stack of three 10 nm Hf1−xZrxO2 layers was deposited that were separated by 0.5 nm thick Al2O3 layers to confine the resulting crystallites within each 10 nm Hf1−xZrxO2 layer. Structural results were later compared to 10 nm Hf1−xZrxO2 layers to confirm that the multi-interlayer stack preserved a comparable structure to the 10 nm thick films (see in the supplementary material). In parallel, measurements were also performed on a 30 nm Hf1−xZrxO2 film without an interlayer.
通常,Hf1-xZrxO2 层中的最佳铁电性能是在 5 至 25 nm 的薄膜厚度范围内报告的。25 由于拉曼强度在此厚度范围内非常弱,因此沉积了三个 10 nm Hf1-xZrxO2 层的层堆栈,这些层由 0.5 nm 厚的 Al2O3 层隔开,以将所得微晶限制在每个 10 nm Hf1-xZrxO2 层内。后来将结构结果与 10 nm Hf1−xZrxO2 层进行比较,以确认多层层堆栈保留了与 10 nm 厚膜相当的结构(参见补充材料)。同时,还对没有夹层的 30 nm Hf1-xZrxO2 薄膜进行了测量。
To identify the phase compositions in the series of fabricated capacitor stacks, a GIXRD analysis was conducted (Fig. 5). Here, “Zr/Hf composition” is referred to as the ratio of the Zr metal-organic precursor to the total number of cycles in the supercycle, described as Zr/(Zr + Hf). Since ZrO2 and HfO2 have a similar growth per cycle, the Zr/(Zr + Hf) cycle ratio is expected to be very similar to the ZrO2 content in the mixed Hf1-xZrxO2 layer. First, the film stacks with Al2O3 interlayers are evaluated. As can be seen in Fig. 5(a), undoped hafnia predominantly crystallizes in the monoclinic phase, whose peaks are represented as m(–111) and m(111) for 2θ values of 28.2° and 31.6°. In this case, the peak intensities for the orthorhombic o(111) and tetragonal phases t(101) at 30.4° and 30.8° are relatively weak, indicating a lower presence of these phases. As the zirconium content increases, the monoclinic peaks reduce, and the reference peak for o(111)/t(101) planes at a 2θ value of about 30.6° becomes the sole diffraction peak, confirming o/t phase stabilization.
为了确定一系列制造的电容器堆栈中的相位组成,进行了 GIXRD 分析(图 5)。在这里,“Zr/Hf 成分”是指 Zr 金属有机前驱体与超循环总次数的比率,称为 Zr/(Zr + Hf)。由于 ZrO2 和 HfO2 在每个周期中具有相似的增长,因此预计 Zr/(Zr + Hf) 循环比与 Hf1-xZrxO2 混合层中的 ZrO2 含量非常相似。首先,评估了具有 Al2O3 夹层的薄膜堆栈。如图 5(a) 所示,未掺杂的铫状体主要在单斜相中结晶,其峰表示为 m(–111) 和 m(111),2θ 值为 28.2° 和 31.6°。在这种情况下,正交相 o(111) 和四方相 t(101) 在 30.4° 和 30.8° 处的峰值强度相对较弱,表明这些相的存在较低。随着锆含量的增加,单斜峰减小,2θ 值约为 30.6° 的 o(111)/t(101) 平面的参考峰成为唯一的衍射峰,证实了 o/t 相位稳定。
GIXRD patterns of 30 nm Hf1−xZrxO2 films deposited with different Zr/Hf content (a) laminate with two alumina interlayers deposited after every 10 nm deposition of the mixed oxide and (b) without interlayers.
沉积具有不同 Zr/Hf 含量的 30 nm Hf1−xZrxO2 薄膜的 GIXRD 图谱 (a) 混合氧化物每 10 nm 沉积后沉积两个氧化铝夹层的层压板,以及 (b) 无夹层。
GIXRD patterns of 30 nm Hf1−xZrxO2 films deposited with different Zr/Hf content (a) laminate with two alumina interlayers deposited after every 10 nm deposition of the mixed oxide and (b) without interlayers.
In addition to the peak intensities, peak positions also play an important role in phase identification. Two independent phenomena are expected to contribute to the shifting of these peak positions when moving from undoped hafnia to undoped zirconia: the left shift arises from the unit cell volume expansion due to different ionic radii of Hf4+ and Zr4+,26 and the right shift of the o(111)/t(101) peak is due to a stronger t-phase stabilization.27 These opposing effects result in an overall irregular peak shifting, as can be seen in Fig. 5(a). In the mixed-oxide samples containing alumina interlayers [Fig. 5(a)], a strong right shift can be observed when transitioning from ZrO2 content of 50%–75%, implying a relevant increase in the t-phase fraction. However, a net left shift is seen for the pure zirconia sample, probably due to the stronger influence of the unit cell volume expansion in this case. The results obtained in this work were also compared to the recent findings of Alcala et al.,26 who analyzed 10 nm thin Hf1−xZrxO2 films with various compositions. It can be noted that similar trends in peak shifting with increasing ZrO2 content can be observed in both 10 nm (Fig. 1 in the supplementary material) and 30 nm thin Hf1−xZrxO2 films. Due to a higher Hf1−xZrxO2 layer thickness, the m-phase fraction increases up to equal Hf/Zr content. This effect was explained by a larger grain size without interlayers and less surface and interface energy impact on the phase stabilization.27
除了峰强度外,峰位置在物相鉴定中也起着重要作用。当从未掺杂的哈夫尼亚移动到未掺杂的氧化锆时,预计两种独立的现象会导致这些峰位置的偏移:左移是由于 Hf4+ 和 Zr4+,26 的离子半径不同而导致的晶胞体积膨胀,而 o(111)/t(101) 峰的右移是由于更强的 t 相稳定性。27 这些相反的效应导致整体不规则的峰移,如图 5(a) 所示。在含有氧化铝夹层的混合氧化物样品中 [图 5(a)],当从 50%–75% 的 ZrO2 含量转变时,可以观察到强烈的右移,这意味着 t 相分数的相关增加。然而,纯氧化锆样品出现净左移,这可能是由于在这种情况下晶胞体积膨胀的影响更大。这项工作获得的结果还与 Alcala 等人26 的最新发现进行了比较,他们分析了具有不同成分的 10 nm 薄 Hf1-xZrxO2 薄膜。可以注意到,在 10 nm(补充材料中的图 1)和 30 nm 薄 Hf1−xZrxO2 薄膜中都可以观察到随着 ZrO2 含量增加而发生的峰偏移的类似趋势。由于较高的 Hf1−xZrxO2 层厚度,m 相分数增加至相等的 Hf/Zr 含量。这种效应的解释是没有夹层的晶粒尺寸较大,对相稳定性的表面和界面能量影响较小。27
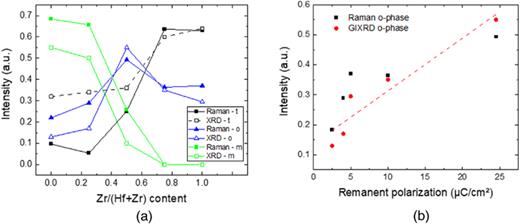
To get a rough estimate of o/t-phase contributions, a Gaussian fit of the obtained GIXRD pattern was performed using the peak positions, peak widths, and peak intensities to estimate the relative phase fractions of the Hf1−xZrxO2 capacitor stacks [Fig. 10(a)]. The most critical part of this fit was distinguishing between the o- and t-phase for later comparison with Raman results. Based on the GIXRD peaks alone, the two phases cannot be distinguished in thin films. Under typical tensile stress conditions of about 2 GPa,28 a 2θ peak of the o-phase can be assumed at 30.4° and a peak of the t phase at 30.8°. Nonetheless, stress and strain can have a strong impact on the peak position. For further analysis, a similar stress/strain needs to be assumed for the complete concentration range. The corresponding results of relative phase fractions with increasing ZrO2 content are plotted in Fig. 10(a). It can be seen that undoped hafnia has the largest m-phase fraction.
为了粗略估计 o/t 相位贡献,使用峰位置、峰宽和峰强度对获得的 GIXRD 模式进行高斯拟合,以估计 Hf1−x ZrxO2 电容器堆栈的相对相位分数 [图 10(a)]。这种拟合最关键的部分是区分 o 期和 t 期,以便以后与拉曼结果进行比较。仅根据 GIXRD 峰,无法在薄膜中区分这两个相。在大约 2 GPa,28 的典型拉伸应力条件下,可以假设 o 相的 2θ 峰值位于 30.4°,t 相的峰值位于 30.8°。尽管如此,应力和应变会对峰位产生很大影响。为了进一步分析,需要假设整个浓度范围具有类似的应力/应变。随着 ZrO2 含量的增加,相对相分数的相应结果如图 10(a) 所示。由此可见,未掺杂的 hafnia 具有最大的 m 相分数。
With increasing Zr concentration, the m-phase is converted to the o- and t-phase, with the o-phase fraction being most significant in the mixed oxide layer for equal amounts of hafnia and zirconium (ZrO2 content 50%). A further increase of the Zr content leads to stabilization of the t-phase. The most robust increase in the t-phase fraction is observed during the transition from a ZrO2 content of 50%–75%.
随着 Zr 浓度的增加,m 相转化为 O 相和 t 相,对于等量的锆和锆(ZrO2 含量 50%),O 相分数在混合氧化层中最为显着。Zr 含量的进一步增加导致 t 相的稳定。在 ZrO2 含量从 50%–75% 的转变过程中观察到 t 相分数的最稳健增加。
A similar trend was observed for the Hf1−xZrxO2 film stack without Al2O3 interlayers [Fig. 5(b)]. For undoped 30 nm HfO2, the m-phase is dominant with only a minor presence of o/t-phases. For almost the complete Zr/Hf composition range, the m-phase content remains higher compared to films with interlayers until only the o/t-phase is detected for 30 nm undoped ZrO2. Interestingly, a side peak at 31° appears, which could not be sufficiently explained so far. Xu and Schenk et al. saw a similar feature for ALD-based 45 nm ZrO229 and CSD-based doped ZrO2 films,30 also without clear phase assignment.
在没有 Al2O3 夹层的 Hf1−xZrxO2 薄膜堆栈中也观察到了类似的趋势 [图 5(b)]。对于未掺杂的 30 nm HfO2,m 相占主导地位,只有少量的 o/t 相存在。对于几乎完整的 Zr/Hf 组成范围,与具有夹层的薄膜相比,m 相含量保持较高,直到仅检测到 30 nm 未掺杂 ZrO2 的 o/t 相。有趣的是,在 31° 处出现了一个侧峰,到目前为止还无法充分解释。Xu 和 Schenk 等人在基于 ALD 的 45 nm ZrO229 和基于 CSD 的掺杂 ZrO2 薄膜中看到了类似的特征,30 也没有明确的相位分配。
The main focus of this study is to differentiate between the o- and t-phase because GIXRD cannot unambiguously distinguish between these two phases but already allows a clear distinction between the o/t phase and the m-phase. Films without an Al2O3 interlayer had a higher m-phase content, so Raman analysis was primarily focused on Hf1−xZrxO2 with interlayers. Here, the smaller grain size is expected to reduce the m-phase content.27
本研究的主要重点是区分 o 期和 t 期,因为 GIXRD 无法明确区分这两个阶段,但已经允许明确区分 o/t 期和 m 期。没有 Al2O3 夹层的薄膜具有较高的 m 相含量,因此拉曼分析主要集中在带有夹层的 Hf1−xZrxO2 上。在这里,较小的晶粒尺寸有望降低 m 相含量。27
Although Gaussian deconvolution of the o/t phase peak in GIXRD may be inaccurate due to the possible impact of stress or strain, at least a linear relationship between the extracted XRD o/t/m phase fraction and the remanent polarization has been confirmed in previous work.31 To provide a similar frame of reference for the films in this investigation, dynamic hysteresis measurements were conducted for Hf1−xZrxO2 capacitors for the same set of structures as used for GIXRD measurements. Figure 6 shows the corresponding polarization-electric field (P-E) curves obtained after applying 12 V triangular pulses. As expected, capacitors show optimum ferroelectric characteristics with an open hysteresis at identical concentrations of Hf and Zr, due to the highest o-phase portions associated with them [as shown in Fig. 10(a)]. Increasing Hf concentration witnesses a monotonic decrease in Pr until an almost paraelectric behavior is reached in undoped HfO2, predominately due to m-phase stabilization. In the other compositional direction, increasing Zr concentration produces a pinching effect in the P-E loops at around 0 MV/cm, which can be correlated to the anti-ferroelectric-like behavior observed due to enhanced t-phase stabilization. The t-phase exerts depolarization fields which reduce Pr at low field conditions, thereby causing pinching of the P-E loops.10 Several other factors also contribute to pinched hysteresis loops, which have been discussed in detail elsewhere.5
尽管由于应力或应变的可能影响,GIXRD 中 o/t 相峰的高斯反卷积可能不准确,但至少提取的 XRD o/t/m 相分数与剩余极化之间存在线性关系已在以前的工作中得到证实。31 为了给本研究中的薄膜提供类似的参考框架,对 Hf1-xZrxO2 电容器进行了动态磁滞测量,这些电容器与用于 GIXRD 测量的相同结构集。图 6 显示了施加 12 V 三角脉冲后获得的相应极化-电场 (P-E) 曲线。正如预期的那样,电容器在相同浓度的 Hf 和 Zr 下表现出最佳的铁电特性和开路磁滞,因为与它们相关的 o 相部分最高 [如图 10(a) 所示]。Hf 浓度的增加见证了 P 的单调降低,直到未掺杂的 HfO2 达到几乎顺电的行为,这主要是由于 m 相稳定。在另一个成分方向上,增加 Zr 浓度在 P-E 回环中产生约 0 MV/cm 的挤压效应,这可能与由于增强的 t 相稳定性而观察到的反铁电样行为有关。t 期施加去极化场,在低场条件下降低 P,从而导致 P-E 环的挤压。10 其他几个因素也会导致磁滞回线收缩,这在其他地方已经详细讨论过。5
However, at higher externally applied fields, the energy is sufficient to overcome these depolarization fields, additionally allowing possible t- to o- phase transitions.10 An even higher electric field is required to induce polarization switching for undoped zirconia compared to the 75% ZrO2 content case.
然而,在更高的外部外加场中,能量足以克服这些去极化场,此外还允许可能的 t 相变到 o 相变。10 与 75% ZrO2 含量的情况相比,未掺杂氧化锆需要更高的电场来诱导极化开关。
From the P-E curves, the remanent polarization of pristine capacitors was evaluated and plotted as a function of Zr/Hf composition [Fig. 6(b)]. It was further compared to the Pr values reported for 10 nm thin HZO films fabricated under similar processing conditions as in this work.26 As expected, the highest Pr values were obtained around identical Hf and Zr content in both sets of samples due to the largest o-phase portions. The Pr value in 30 nm thick Hf1−xZrxO2 with interlayers (20.8 μC/cm2) is around the same value as in 10 nm thin Hf1−xZrxO2 (19.1 μC/cm2). The overall Pr similarities in the 10 nm and 30 nm Hf1−xZrxO2 with interlayers further affirm the notion that despite having a larger cumulative thickness, insertion of discrete alumina layers can reproduce roughly thin film behavior.
根据 P-E 曲线,评估了原始电容器的剩余极化,并将其绘制为 Zr/Hf 组成的函数 [图 6(b)]。进一步将其与在与本研究类似的加工条件下制造的 10 nm 薄 HZO 薄膜报告的 P 值进行了比较。26 正如预期的那样,由于两组样品的 O 相部分最大,在相同的 Hf 和 Zr 含量附近获得了最高的 P 值。30 nm 厚的 Hf1-xZrxO2 夹层 (20.8 μC/cm2) 的 P 值与 10 nm 薄的 Hf1-xZrxO2 (19.1 μC/cm2) 中的值大致相同。10 nm 和 30 nm Hf1−xZrxO2 与夹层的总体 P 相似性进一步证实了这样一个概念,即尽管具有较大的累积厚度,但离散氧化铝层的插入可以大致再现薄膜行为。
To verify the crystal phase proportions obtained by fitting of the XRD patterns, Pr was plotted as a function of the o-phase fraction. Figure 10(b) shows Pr of 30 nm Hf1−xZrxO2 samples for all Zr precursor ratios with respect to their corresponding o-phase fractions. As expected, the results show an almost linear trend for all compositions, with the strongest deviations for undoped ZrO2.
为了验证通过拟合 XRD 图谱获得的晶相比例,将 P 绘制为 o 相分数的函数。图 10(b) 显示了所有 Zr 母离子比的 30 nm Hf1−xZrxO2 样品相对于其相应 O 相分数的 P。正如预期的那样,结果显示所有成分都呈近线性趋势,其中未掺杂的 ZrO2 的偏差最大。
(a) Polarization-electric field hysteresis loops for 30 nm Hf1−xZrxO2-based capacitors with an Al2O3 interlayer every 10 nm Hf1−xZrxO2 for different Zr/Hf compositions. (b) Remanent polarization vs Zr content for 10 nm and 30 nm Hf1−xZrxO2-based capacitors with Al2O3 interlayer.
(a) 针对不同的 Zr/Hf 成分,基于 30 nm Hf1-xZrxO2 的电容器的极化-电场磁滞回线,每 10 nm Hf1-xZrxO2 有一个 Al2O3 夹层。(b) 10 nm 和 30 nm Hf1−xZrxO2 基电容器(含 Al2O3 夹层)的剩余极化与 Zr 含量的关系。
(a) Polarization-electric field hysteresis loops for 30 nm Hf1−xZrxO2-based capacitors with an Al2O3 interlayer every 10 nm Hf1−xZrxO2 for different Zr/Hf compositions. (b) Remanent polarization vs Zr content for 10 nm and 30 nm Hf1−xZrxO2-based capacitors with Al2O3 interlayer.
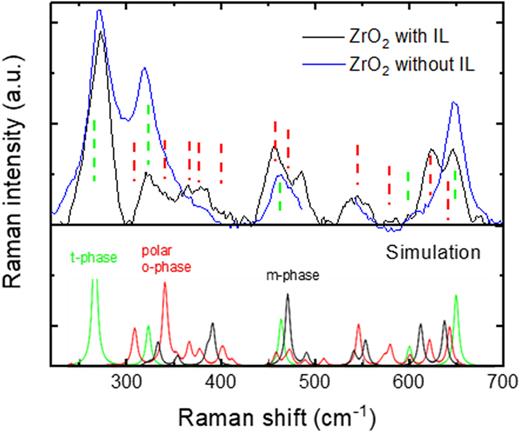
In the next step, Raman spectroscopy (RS) was also used to characterize the Hf1−xZrxO2 structures containing alumina interlayers because of the limitations of GIXRD when it comes to distinguishing the o and t phase, as discussed previously. From the current series of experiments, a simultaneous presence of the three phases was found in a wide compositional range. To further refine the separation of the o-phase from the t-phase, optical characterization with RS was performed to understand the phase evolution with increasing Zr concentration (Fig. 7).
在下一步中,拉曼光谱 (RS) 还用于表征含有氧化铝夹层的 Hf1−xZrxO2 结构,因为如前所述,GIXRD 在区分 o 相和 t 相方面的局限性。从目前的一系列实验中,发现这三相同时存在于很宽的组成范围内。为了进一步优化 o 相与 t 相的分离,使用 RS 进行了光学表征,以了解随着 Zr 浓度的增加而发生的相演变(图 7)。
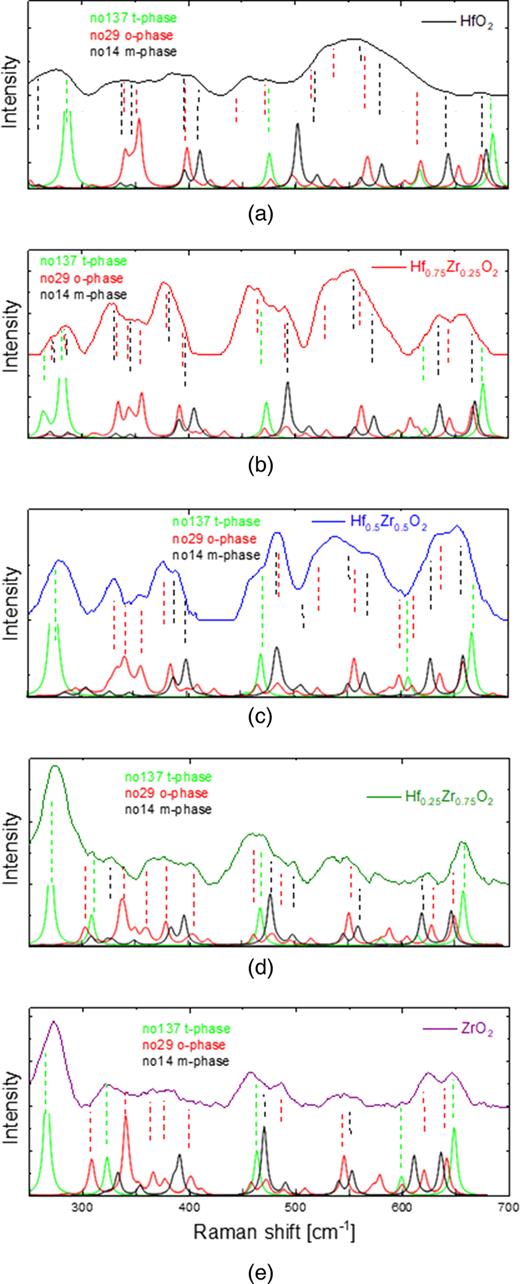
At first glance, no clear trend in the measured spectra is visible, and a direct comparison to the simulated results is necessary. In general, the main trends found in XRD can be confirmed by RS (Fig. 8). To get a better understanding, for all RS measurements, a Gaussian peak fitting was performed for the main peak positions as found by simulation [Fig. 7(b)]. Only the width of the RS peaks was fixed to 17–20 cm−1, while the peak positions themselves were kept floating. After fitting, the most intense m-, o-, and t-phase RS mode, peak positions could be verified in the experimental data, and changes in the peak position nicely fit the expected trends (Fig. 9).
乍一看,测量光谱中没有明显的趋势,需要与模拟结果直接比较。一般来说,在 XRD 中发现的主要趋势可以通过 RS 来确认(图 8)。为了更好地理解,对于所有 RS 测量,对模拟发现的主要峰位置进行了高斯峰拟合 [图 7(b)]。只有 RS 峰的宽度固定为 17-20 cm-1,而峰位置本身保持浮动。拟合后,可以在实验数据中验证最强的 m 、o 和 t 期 RS 模式、峰值位置,并且峰值位置的变化与预期趋势非常吻合(图 9)。
(a) Raman spectroscopy measurements for 30 nm Hf1−xZrxO2-based capacitors with Al2O3 interlayer every 10 nm Hf1−xZrxO2 for different Zr/Hf compositions. (b) Raman spectroscopy measurements for undoped ZrO2 fitted by Gaussian peaks (width 17 cm−1) for the main t- and o-phase Raman modes. A similar fitting was performed for the simulated peak positions from Figs. 9(a) and 9(c). Results are shown in Fig. SI2 in the supplementary material.
(a) 针对不同的 Zr/Hf 组成,对 30 nm Hf1-xZrxO2 基电容器进行拉曼光谱测量,每 10 nm Hf1-xZrxO2 有 Al2O3 夹层。(b) 未掺杂 ZrO2 的拉曼光谱测量,由主要 t 相和 o 相拉曼模式的高斯峰(宽度 17 cm−1)拟合。对图 9(a) 和 9(c) 中的模拟峰位置进行了类似的拟合。结果如图 1 所示。SI2 在补充材料中。
(a) Raman spectroscopy measurements for 30 nm Hf1−xZrxO2-based capacitors with Al2O3 interlayer every 10 nm Hf1−xZrxO2 for different Zr/Hf compositions. (b) Raman spectroscopy measurements for undoped ZrO2 fitted by Gaussian peaks (width 17 cm−1) for the main t- and o-phase Raman modes. A similar fitting was performed for the simulated peak positions from Figs. 9(a) and 9(c). Results are shown in Fig. SI2 in the supplementary material.
Raman spectroscopy measurements for 30 nm Hf1−xZrxO2-based capacitors with Al2O3 interlayers for different Zr/Hf composition in direct comparison to DFT simulated spectra.
与 DFT 模拟光谱直接比较,对 30 nm Hf1−xZrxO2 基电容器进行拉曼光谱测量,其中 Al2O3 夹层具有不同的 Zr/Hf 成分。
Raman spectroscopy measurements for 30 nm Hf1−xZrxO2-based capacitors with Al2O3 interlayers for different Zr/Hf composition in direct comparison to DFT simulated spectra.
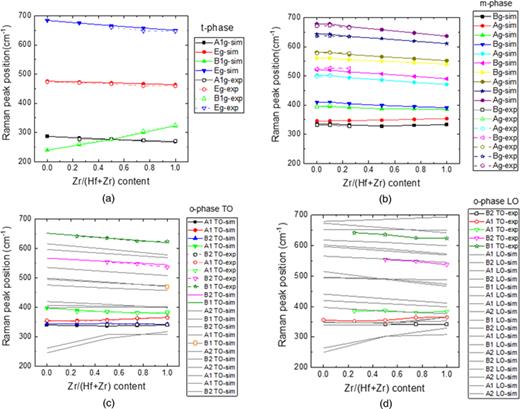
Raman peak positions for different modes for the (a) t-phase (b) m-phase (c) o-phase (TO) (d) o-phase LO—as a function of the Zr/Hf composition for 30 nm Hf1−xZrxO2-based capacitors with Al2O3 interlayers.
(a) t 相 (b) m 相 (c) o 相 (TO) (d) o 相 LO 不同模式的拉曼峰位置 — 作为具有 Al2O3 夹层的 30 nm Hf1−xZrxO2 基电容器的 Zr/Hf 组成的函数。
Raman peak positions for different modes for the (a) t-phase (b) m-phase (c) o-phase (TO) (d) o-phase LO—as a function of the Zr/Hf composition for 30 nm Hf1−xZrxO2-based capacitors with Al2O3 interlayers.
Hf1−xZrxO2 layers with Al2O3 interlayers: (a) Extracted phase portions after Gaussian peak fitting of XRD diffraction peaks from Fig. 5(a). Results are compared to Raman peak fitting results for all three phases. (b) Remanent polarization from Fig. 6(b) as a function of the o-phase content from Fig. 10(a).
Hf1−xZrxO2 层与 Al2O3 夹层:(a) 图 5(a) 中 XRD 衍射峰的高斯峰拟合后提取的相部分。将结果与所有三个固定相的拉曼峰拟合结果进行比较。(b) 图 6(b) 中的剩余极化与图 10(a) 中 o 相含量的函数关系。
Hf1−xZrxO2 layers with Al2O3 interlayers: (a) Extracted phase portions after Gaussian peak fitting of XRD diffraction peaks from Fig. 5(a). Results are compared to Raman peak fitting results for all three phases. (b) Remanent polarization from Fig. 6(b) as a function of the o-phase content from Fig. 10(a).
Interestingly, a mode crossing is visible for
有趣的是,对于 和
Overall, the intensity of the o-phase was weaker compared to the t/m-phase, which is related to the higher amount of phonon modes as confirmed by DFT simulation. A comparison of the fitted peaks indicated a better correlation to the strongest TO-modes of the o-phase and more discrepancies for LO modes [Figs. 9(c) and 9(d)]. In many cases, o- and m-phase peak positions overlapped, and only o-phase mode positions with very minor m-phase contributions are used to determine the o-phase content (at about 340, 360, and 620 cm−1). For an approximation of the phase content in the five layers, the integration of the areas under the peaks of the m-phase positions at about 380, 570, and 640 cm−1 and the t-phase positions at about 320, 460, and 650 cm−1 were used. The peak at about 250 cm−1 also included contributions from the TiN bottom electrode and was excluded from the analysis. The sum of the peak area for one phase was divided by the total peak area for a given film, and the results are plotted in Fig. 10(a) and compared to the GIXRD phase extraction results (for details, see the supplementary material). Similar trends between the two techniques and resulting extractions were observed. A precise phase content cannot be determined by both methods since, in both cases, only the area ratios of some peaks were used, but not the full spectrum of the respective phases.
总体而言,与 t/m 相位相比,o 相位的强度较弱,这与 DFT 模拟证实的声子模式数量较高有关。拟合峰的比较表明,与 o 期最强的 TO 模式的相关性更好,而 LO 模式的差异更大 [图 9(c) 和 9(d)]。在许多情况下,o 期和 m 期峰值位置重叠,并且仅使用 m 期贡献非常小的 o 期模式位置来确定 o 期含量(约为 340、360 和 620 cm−1)。为了近似五层中的相含量,使用了大约 380、570 和 640 cm-1 处的 m 期位置峰值下的面积和大约 320、460 和 650 cm-1 处的 t 期位置下的面积的积分。约 250 cm-1 处的峰也包括来自 TiN 底部电极的贡献,因此被排除在分析之外。将一个相的峰面积总和除以给定薄膜的总峰面积,结果绘制在图 10(a) 中,并与 GIXRD 相萃取结果进行比较(有关详细信息,请参阅补充材料)。观察到两种技术和所得提取之间的相似趋势。两种方法都无法确定精确的相含量,因为在这两种情况下,都只使用了一些峰的面积比,而没有使用相应相的完整谱图。
In summary, a clear distinction between the o- and t-phase is possible for films with high ZrO2 content because only a minor m-phase content is present. For films with joint presence of high m- and o- phase fractions, such as Hf-rich Hf1-xZrxO2 films, only peak positions with little overlap of both phases can be used. So far, in XRD analysis of the peak position at 2Θ = 30.4–30.8°, a constant stress/strain of 1–2 GPa is assumed. For this condition, a peak deconvolution is performed, which indicates a certain o/t phase ratio. A similar assumption was used in this manuscript. The o-phase was assigned to a Gaussian peak at 30.4° and the t-phase to 30.8°, respectively. Using this assumption, a good correlation between the XRD and Raman data was found in Fig. 10(a), especially for high ZrO2 content in the films, where almost no m-phase portion is present. Accordingly, the XRD analysis done at the beginning of the text could be confirmed by Raman as a reasonable approach. Similar to the case of GIXRD, RS results were compared with Pr values. Again, an almost linear trend can be confirmed, with the strongest deviations for undoped ZrO2 [Fig. 10(b)].
总之,对于具有高 ZrO2 含量的薄膜,可以明确区分 o 相和 t 相,因为仅存在少量的 m 相含量。对于同时存在高 m 相和 o 相分数的薄膜,例如富含 Hf 的 Hf1-xZrxO2 薄膜,只能使用两相几乎没有重叠的峰位置。到目前为止,在 2Θ = 30.4–30.8° 处峰位置的 XRD 分析中,假设恒定应力/应变为 1–2 GPa。对于这种情况,执行峰值反卷积,这表明一定的 o/t 相位比。这份手稿中使用了类似的假设。o 期分别分配给 30.4° 处的高斯峰和 30.8° 处的 t 期。利用这个假设,在图 10(a) 中发现了 XRD 和拉曼数据之间的良好相关性,特别是对于薄膜中 ZrO2 含量高的情况,其中几乎没有 m 相部分。因此,在文本开头进行的 XRD 分析可以通过 Raman 确认为一种合理的方法。与 GIXRD 的情况类似,将 RS 结果与 P 值进行比较。同样,可以确认一个几乎线性的趋势,未掺杂的 ZrO2 的偏差最大 [图 10(b)]。
(a) Raman spectroscopy measurements for undoped 30 nm HfO2 with and without Al2O3 interlayer for different anneal conditions. (b) GIXRD measurements on the same sample set for the same anneal conditions.
(a) 在不同退火条件下对无 Al2O3 夹层的未掺杂 30 nm HfO2 进行拉曼光谱测量。(b) 在相同退火条件下对同一样品组进行的 GIXRD 测量。
(a) Raman spectroscopy measurements for undoped 30 nm HfO2 with and without Al2O3 interlayer for different anneal conditions. (b) GIXRD measurements on the same sample set for the same anneal conditions.
The undoped HfO2 and ZrO2 systems (Figs. 11 and 12) are evaluated in the next section with and without Al2O3 interlayers. The undoped HfO2 with Al2O3 interlayers did not completely crystallize during 500 °C 20 s N2 anneals, as already mentioned earlier. GIXRD shows a broad diffuse diffraction structure, as expected for small nano-crystallites. A first 20 s long 700 °C anneal increased m- and o/t reflections, which became even stronger after a second 700 °C anneal for 20 s. In contrast, the 30 nm HfO2 film without interlayers indicates a predominately m-phase pattern with only minor o/t-phase contributions [Fig. 11(b)].
下一节将评估未掺杂的 HfO2 和 ZrO2 体系(图 11 和 12),有和没有 Al2O3 夹层。如前所述,具有 Al2O3 夹层的未掺杂 HfO2 在 500 °C 20 s N2 退火过程中没有完全结晶。GIXRD 显示出宽泛的漫射衍射结构,正如小纳米晶所预期的那样。第一次 20 秒长的 700 °C 退火增加了 m- 和 o/t 反射,在第二次 700 °C 退火 20 秒后变得更强。相比之下,没有夹层的 30 nm HfO2 薄膜表明主要是 m 期图案,只有很小的 o/t 期贡献 [图 11(b)]。
A similar trend is visible in Raman spectroscopy [Fig. 11(a)]. A 500 °C anneal showed no HfO2 related signal for HfO2 with interlayers, but m- and o-phase modes became visible after the two 700 °C anneals. In contrast, all m-phase modes became clearly present for the film without interlayers, and only small intense o-phase modes are indicated. In addition, t-phase modes do not appear in the 30 nm thick continuous HfO2 films. Accordingly, the GIXRD peak at a 2θ value of about 30.4 can be mainly attributed to the o-phase and is only seen in the HfO2 films with interlayers.
在拉曼光谱中也可以看到类似的趋势 [图 11(a)]。500 °C 退火显示 HfO2 与夹层没有 HfO2 相关信号,但在两次 700 °C 退火后,m 期和 o 期模式变得可见。相比之下,没有夹层的薄膜的所有 m 期模式都变得清晰存在,并且只显示了小的强烈 o 期模式。此外,t 期模式不会出现在 30 nm 厚的连续 HfO2 薄膜中。因此,2θ 值约为 30.4 的 GIXRD 峰主要归因于 o 相,并且仅在具有夹层的 HfO2 薄膜中可见。
An analogous comparison can also be performed for ZrO2 with and without Al2O3 interlayers. Looking at the GIXRD pattern in Figs. 5(a) and 5(b), only o/t-phase features are present. The smaller full-width-half-maximum (FWHM) for ZrO2 without interlayer allows to resolve the peak as a double-peak pattern with peak positions at about 2θ = 30.6° and 31°. This double-peak could possibly also be present in the ZrO2 with interlayers but cannot be resolved due to the enhanced FWHM. As often reported, amorphous Al2O3 interlayers in ZrO2 separate the complete ZrO2 in thinner films with smaller grain size.20 Since this separation is not present in the film without interlayers, larger grains and a smaller FWHM in XRD are expected. Using the Debye-Scherrer equation,32 an average grain size of 7 nm for the film with interlayers compared to 12 nm for the unseparated ZrO2. Comparison to simulated XRD patterns indicates that the right-side shoulder at 31.0° could possibly be attributed to the anti-polar oI-phase (space group Pbca). If this shoulder would come from the anti-polar oI-phase, an additional reflection needs to be present at 29.4°, which could not be detected. Accordingly, the origin of the shoulder cannot be clarified in detail.
也可以对有和没有 Al2O3 夹层的 ZrO2 进行类似的比较。查看图 5(a) 和 5(b) 中的 GIXRD 模式,仅存在 o/t 相特征。对于没有夹层的 ZrO2,较小的全宽半最大值 (FWHM) 允许将峰解析为双峰模式,峰位置约为 2θ = 30.6° 和 31°。这个双峰也可能存在于带有夹层的 ZrO2 中,但由于 FWHM 增强而无法解析。正如经常报道的那样,ZrO2 中的无定形 Al2O3 夹层将完整的 ZrO2 分离成晶粒尺寸更小的较薄薄膜。20 由于没有夹层的薄膜中不存在这种分离,因此预计 XRD 中会出现较大的晶粒和较小的 FWHM。使用德拜-谢勒方程32,有夹层的薄膜的平均晶粒尺寸为 7 nm,而未分离的 ZrO2 的平均晶粒尺寸为 12 nm。与模拟的 XRD 图谱进行比较表明,31.0° 的右侧肩部可能归因于反极 oI 期(空间群 Pbca)。如果这个肩部来自反极 oI 阶段,则需要在 29.4° 处出现额外的反射,这是无法检测到的。因此,无法详细阐明肩部的来源。
In the next step, RS was performed on the same sample set (Fig. 12). As discussed in Fig. 8(e), the ZrO2 film with interlayer consists of about two-thirds t-phase and one-third o-phase contributions. When no interlayer is present, predominately t-phase features are detected, and only very minor polar can be determined. Still, no m-phase is detected within the resolution limit of RS.
下一步,对同一样品组进行 RS 检测(图 12)。如图 8(e) 所示,带有夹层的 ZrO2 薄膜由大约三分之二的 t 相和三分之一的 o 相贡献组成。当不存在夹层时,主要检测到 t 期特征,并且只能确定非常小的极性。尽管如此,在 RS 的分辨率限内仍未检测到 m 期。
Raman spectroscopy measurements for undoped 30 nm ZrO2 with and without Al2O3 interlayer.
未掺杂 30 nm ZrO2 的拉曼光谱测量,带和不带 Al2O3 夹层。
Raman spectroscopy measurements for undoped 30 nm ZrO2 with and without Al2O3 interlayer.
In both cases, for undoped HfO2 and ZrO2, the thicker films without interface lead to larger grains and, with this, a cleaner phase formation of the m- or t-phase, respectively. Introducing an Al2O3 interlayer reduces the grain size and causes an additional o-phase content. These results fit nicely with former DFT predictions by Materlik et al.,27 where the m-phase is predicted for larger HfO2 grains with an increased o-phase probability for smaller grains. For ZrO2 grains, the t-phase is the dominant phase for small grains, and the o-phase becomes more likely for an intermediate grain size of 20–30 nm before the m-phase is again the main phase for larger grains. Theory explains the free energy of the grains from a positive bulk contribution, which increases from m- over o- to the t-phase, reduced by a surface/interface contribution. In HfO2 the bulk t-phase is much more unfavorable than in ZrO2. The surface/interface contributions are similarly large in HfO2 and ZrO2, with increasing importance for smaller grains. Comparing HfO2 and ZrO2 grains and reducing the grain size, the o-phase is found for intermediate size. But in HfO2 the size range where the t-phase is stable is moved to a very small grain size.
在这两种情况下,对于未掺杂的 HfO2 和 ZrO2,没有界面的较厚薄膜会导致更大的晶粒,从而分别形成更干净的 m 相或 t 相。引入 Al2O3 中间层可减小晶粒尺寸并导致额外的 o 相含量。这些结果与 Materlik 等人之前的 DFT 预测非常吻合,27 其中预测较大的 HfO2 晶粒的 m 相,而较小晶粒的 o 相概率增加。对于 ZrO2 晶粒,t 相是小晶粒的主相,在 m 相再次成为较大晶粒的主相之前,20-30 nm 的中间晶粒尺寸更有可能出现 O 相。理论解释了来自正体积贡献的晶粒的自由能,该贡献从 m- 超过 o- 增加到 t 相,因表面/界面贡献而减少。在 HfO2 中,本体 t 相比在 ZrO2 中更不利。HfO2 和 ZrO2 的表面/界面贡献同样大,对于较小的晶粒越来越重要。比较 HfO2 和 ZrO2 晶粒并减小晶粒尺寸,发现中等尺寸的 o 相。但在 HfO2 中,t 相稳定的尺寸范围被移动到非常小的晶粒尺寸。
IV. SUMMARY IV. 概述
The combination of XRD and Raman spectroscopy enabled a clear identification of the non-polar monoclinic and tetragonal, or the polar and anti-polar orthorhombic phases in mixed hafnium–zirconium oxide films. Especially for the undoped ZrO2 case, where so far Rietveld refinement of XRD patterns gave only arbitrary phase determination, now Raman spectroscopy allows an unambiguous differentiation of both structures. Following tetragonal phase phonon modes with Hf/Zr composition indicated a crossing of
XRD 和拉曼光谱的结合能够清楚地识别混合铪-氧化锆薄膜中的非极性单斜相和四方相,或极性和反极性正交相。特别是对于未掺杂的 ZrO2 情况,到目前为止,XRD 图谱的 Rietveld 精修只能提供任意的相位测定,现在拉曼光谱允许明确区分两种结构。在具有 Hf/Zr 成分的四方相位声子模式之后,表明 和
SUPPLEMENTARY MATERIAL 补充材料
See the supplementary material for the additional XRD data to characterize the layer stack compared to the single-layer thin film. Furthermore, additional details of the simulation results are reported: structural results for the investigated supercells and detailed results for the obtained Raman mode frequencies and their symmetry assignment.
有关其他 XRD 数据,请参阅补充材料,以表征与单层薄膜相比的层堆栈。此外,还报告了仿真结果的其他细节:所研究的超级胞的结构结果以及获得的拉曼模式频率及其对称性分配的详细结果。
ACKNOWLEDGMENTS 确认
The authors like to thank M. Deluca and M. Popov for fruitful discussions. P.D.L. and B.X. were financially supported by the Deutsche Forschungs Gemeinschaft DFG within the following projects [Zeppelin (No. 433647091) and Homer (No.430054035)]. M.M. would like to thank Sony Corporation for funding. A.K. gratefully acknowledges the Gauss Centre for Supercomputing e.V. (www.gauss-centre.eu) for funding this project by providing computing time on the GCS Supercomputer SuperMUC-NG at Leibniz Supercomputing Centre (www.lrz.de) under Grant No. pr27su. Parts of this work was financially supported out of the state budget approved by the delegates of the Saxon State parliament.
作者感谢 M. Deluca 和 M. Popov 进行的富有成效的讨论。P.D.L. 和 B.X. 在以下项目中得到了 Deutsche Forschungs Gemeinschaft DFG 的财政支持[Zeppelin(第 433647091 号)和 Homer(第 430054035 号)]。M.M. 衷心感谢 Sony Corporation 的资助。A.K. 非常感谢高斯超级计算中心 e.V. (www.gauss-centre.eu) 资助该项目,在莱布尼茨超级计算中心 (www.lrz.de) 的 GCS 超级计算机 SuperMUC-NG 上提供计算时间,资助号为。pr27su 的。这项工作的一部分由撒克逊州议会代表批准的国家预算提供财政支持。
AUTHOR DECLARATIONS 作者声明
Conflict of Interest 利益冲突
The authors have no conflicts to disclose.
作者没有要披露的冲突。
Author Contributions 作者贡献
Uwe Schroeder: Conceptualization (equal); Data curation (equal); Formal analysis (equal); Funding acquisition (equal); Writing – original draft (equal); Writing – review & editing (equal). Ridham Sachdeva: Formal analysis (equal); Investigation (lead); Writing – original draft (supporting). Patrick D. Lomenzo: Formal analysis (supporting); Investigation (equal); Resources (equal); Writing – review & editing (equal). Bohan Xu: Formal analysis (supporting); Investigation (equal). Monica Materano: Formal analysis (supporting); Investigation (equal). Thomas Mikolajick: Funding acquisition (equal); Project administration (lead); Writing – review & editing (equal). Alfred Kersch: Funding acquisition (equal); Resources (equal); Software (equal); Writing – original draft (equal); Writing – review & editing (equal).
乌韦·施罗德:概念化(相等);数据管理(相等);形式分析(相等);资金获取(相等);Writing – 原始草稿 (相等);写作 - 审查和编辑(平等)。里德姆·萨奇德瓦:形式分析(相等);调查(牵头);写作 – 原始草稿(支持)。帕特里克·洛门佐:形式分析(支持);调查 (相等);资源(相等);写作 - 审查和编辑(平等)。徐博涵:形式分析(支持);调查 (等于)。莫妮卡·马特拉诺:形式分析(支持);调查 (等于)。托马斯·米科拉吉克:资金获取(相等);项目管理(牵头);写作 - 审查和编辑(平等)。阿尔弗雷德·克什:资金获取(相等);资源(相等);软件(相等);Writing – 原始草稿 (相等);写作 - 审查和编辑(平等)。
DATA AVAILABILITY 数据可用性
The data that support the findings of this study are available from the corresponding author upon reasonable request.
支持本研究结果的数据可应合理要求从通讯作者处获得。
REFERENCES 引用
99, 102903 (2011).https://doi.org/10.1063/1.3634052
99(11), 112901 (2011).https://doi.org/10.1063/1.3636417